I’m allways astonished by how many function seemingly have nothing to do with circles and yet somehow a pi managed to snuck itself in
Usually when that happens there’s a way to tie it back to circles, but it’s not always easy to find
You could say you just go round and round hunting for it, but no matter how hard you try you just can’t corner it.
Well, you could.
there’s a way to tie it back to circles
Not necessarily circles, but conic sections. When you take a series of a fixed exponent over a variable x, and graph it, that graph is a parabola.
A parabola is a slice through a cone. Tada, pi appears.
It makes sense if you consider that mass without outside forces forms a sphere. If you have enough atoms you get a sphere, and if you only have two they will circle eachother. Two hydrogen atoms are two spheres of neutrons and protons being circled by an electron circling eachother. It’s circles all tge way down.
tbf, the 2nd sum is exactly the first one just multiplied by 1/2. though i get that the progression is natural, even, and odd.
the last one is definitely
oddpuzzling, but i cannot intuitively get the first one. how does summing the inverse of triangular number equal 2?I believe starting with 1/1 which equals 1, you are then adding infinitely (fractions) on top of the 1. So 1, then 1 1/2, ect, so the next full integer to be hit (infinitely down the line) would be 2.
I don’t do high level math so I hope this explanation is correct or intelligible, this is just how I understand it intuitively
But the first few values are:
1 + 1/3 + 1/6 + 1/10 + 1/15 + 1/21 + 1/28…
I really don’t see any pattern there showing why it converges to 2 exactly
Edit:
After thinking some more, you could write the sum as:
(Sum from n=1 to infinity of): 2/(n * (n + 1))
That sum is smaller than the sum of:
2 * (1/n2) which converges to π2/3
So I can see why it converges, just not where to.
I didn’t see the pattern either and had to look it up. Apparently, you can rewrite 1 + 1/(1+2) + 1/(1+2+3)+… as 2(1 - 1/2 + 1/2 - 1/3 +…+1/n - 1/(n + 1)) = 2(1 - 1/(n + 1))
From there, the limit of 2 is obvious, but I guess you just have to build up intuition with infinite sums to see the reformulation.
So the amount you are adding is getting smaller with each iteration, 1/4 is smaller than 1/2, however you are still adding 1/4 on top of the 1/2, and those two are combined, closer to “1” than either of them independently correct? (1/2 +1/4 =1/3. 1/3>1/2)
So if the number gets bigger forever than at some point it will eventually hit “1”, since we already started with “1” the next “1” will be “2”
I hope I’m explaining it well enough, it’s similar to how 3.33(repeating)x3…=10 (though technically for different reasons)
deleted by creator
Those add to 1.75, just keep adding (infinitely)
It’s not saying the proof is obvious, just that the result is plausible on its face.
1/(n * (n+1)) = 1/n - 1/(n+1)
1/(1 * 2) + 1/(2 * 3) + 1/(3 * 4) + … = 1 - 1/2 + 1/2 - 1/3 + 1/3 - 1/4 + … = 1
Written a bit more explicitly (although I kinda handwaved away the final term–the point is that you end up with one unpaired term which goes to zero)
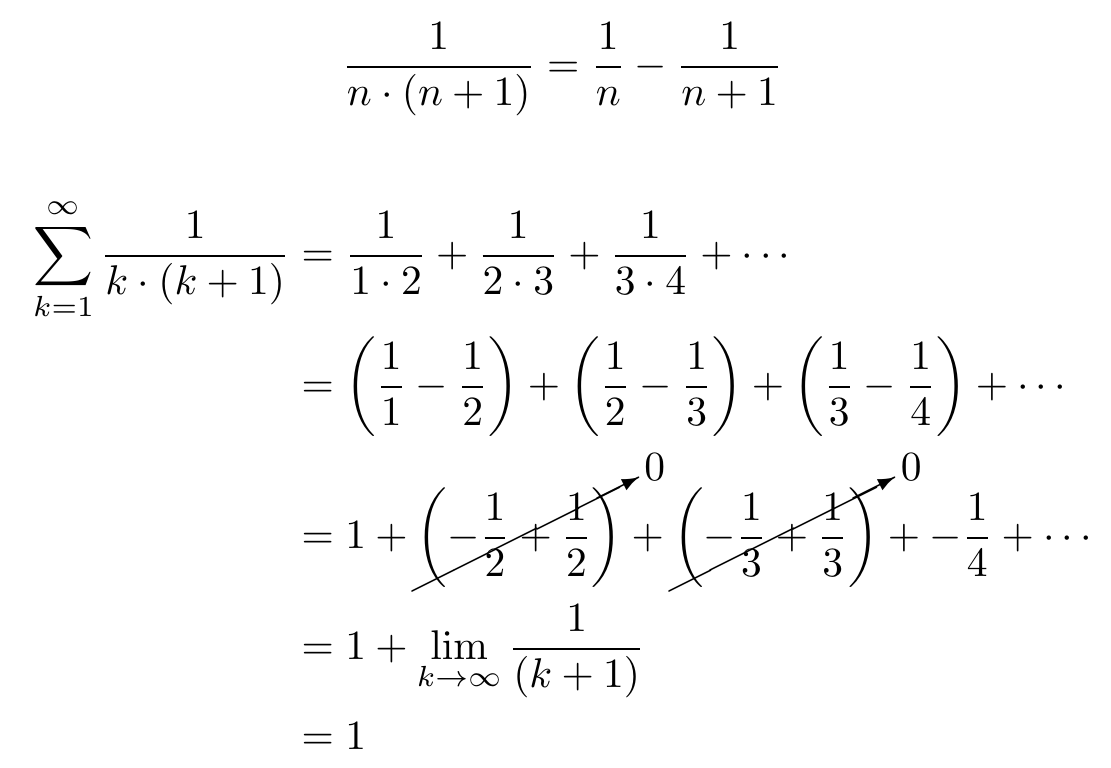
edit: I was honestly confused about how exactly this related to the question, but seeing the comment from @yetAnotherUser@discuss.tchncs.de (not visible from Hexbear) which showed that the first sum in the image is equivalent to
the sum from n = 1 to ∞ of 2/(n * (n + 1))
made things clear (just take the above, put 2 in the numerator, and you get a result of 2)
1 + 2 + 3 + 4 + 5 + … = -1/12
Under very special circumstances an argument can be made for this, at least
Not really. The left side comes from trying to evaluate the Reimann zeta function at a point outside its domain, and the right side comes from evaluating the analytic continuation of the zeta function at the same point. The deepest truth it reveals is that applying the definition of a function outside its domain can give you nonsense results.
It’s one of those ideas like Scrödinger’s cat or pulling yourself up by your bootstraps, where something that was intended to illustrate an absurdity is instead taken as representing some amazing and unintuitive truth.
Never understood those circumstances, but I choose to believe that all even numbers summed are -2/12 and all odd numbers therefore 1/12.
That’s where I assumed it was going.
What’s the big deal? Just solve for …
… = (π^2 / 6) - (1 + 1/4 + 1/9)
Ez
/s
Tired of waiting 2 seconds for hyperpi to download? Simply do infinity calculations by hand and some basic algebra. Cut out the middle man. Thanks, internet!
Yeah, but is the function for 1 over odd numbers, or 1 over prime numbers …
1 isn’t a prime number
And 2 is.
how dare you post math in this sub
omg I missed the … and was confused as heck




